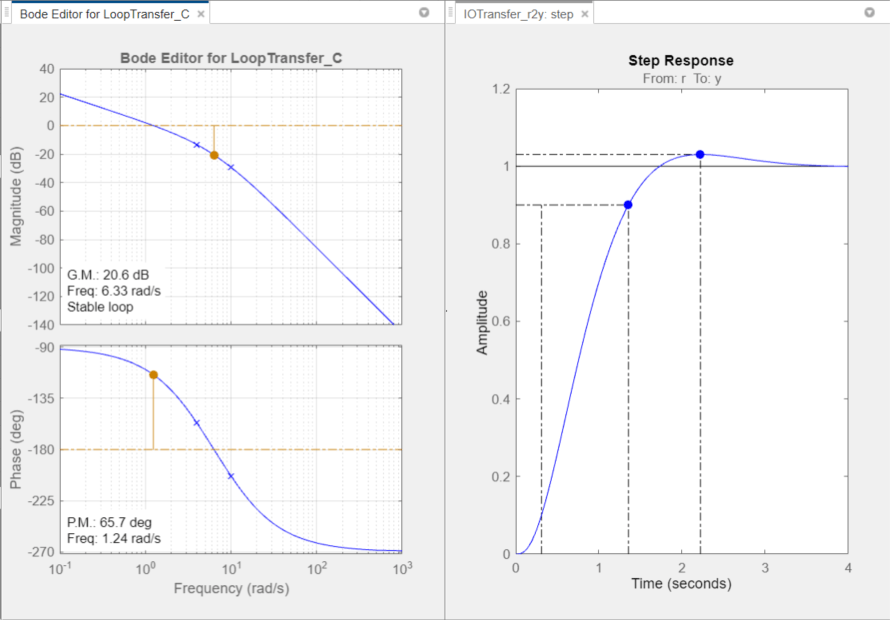
What we want, though, is Z, the number of zeros in the right half plane. If we perform a mapping (as explained on the previous page) of the function '1+L(s)' with a path in 's' that encircle the entire right half plane and we count the encirclements of the origin in the '1+L(s)' domain in the clockwise direction we get the number N=Z-P (where Z is the number of zeros, and P is the number of poles). Response of a transfer function with poles in the right half plane grows Has any zeros in the right half of the s-plane (recall that the natural Transfer function (which is the characteristic equation of the system) This is equivalent to asking whether the denominator of the We would like to be able to determine whether or not the closed loop system, The Nyquist Path (with poles on jω axis).
Determining Stability using the Nyquist Plot